Mohr's circle & Principle Stresses

It is a useful graphical technique for finding principal stresses and strains in materials. Mohr’s circle also tells you the principal angles (orientations) of the principal stresses without your having to plug an angle into stress transformation equations. The Mohr circle is then used to determine graphically the stress components acting on a rotated coordinate system, i.e., acting on a differently oriented plane passing through that point.

There will be one plane on which the normal stress value is maximum, this plane is known as the Principal plane ( more precisely the maximum principal plane) and normal stress on this plane is known as principal stress (more precisely maximum principal stress). Similarly, there will be one more plane on which the normal stress value is minimum, this is also a principal plane (minimum principal plane), and normal stress on this plane is known as Principal stress (minimum principal stress).

Starting with a stress or strain element in the XY plane, construct a grid with normal stress on the horizontal axis and shear stress on the vertical. (Positive shear stress plots at the bottom.) Then just follow these steps:

• Plot the vertical face coordinates V(σxx, τxy).
• Plot the horizontal coordinates H(σyy, –τxy).
• Draw a diameter line connecting Points V (from Step 1) and H (from Step 2).
• Sketch the circle around the diameter from Step 3.
• Compute the normal stress position for the circle’s center point (C).
• Calculate the radius (R) for the circle.
• Determine the principal stresses σP1 and σP2.
• Compute the principal angles ΘP1 and ΘP2.

Mohr's circle and principle stresses are concepts used in the field of mechanics and engineering to analyze and understand the stress state of a material or structure. These concepts were developed by the German engineer Christian Otto Mohr in the 19th century.

Mohr's Circle:

Mohr's circle is a graphical representation that helps visualize the stress components acting on a material at a specific point. It is constructed by plotting the normal stress (σ) on the x-axis and the shear stress (τ) on the y-axis. The circle is drawn with its center located at the average normal stress (σ_avg) and its radius equal to half the difference between the maximum and minimum normal stresses (σ_max - σ_min) acting on the material.

By rotating the circle around its center, it is possible to determine the principal stresses and the corresponding planes of maximum and minimum shear stress. The principal stresses are the normal stresses acting on the planes where shear stress is zero. These planes are called principal planes.

Principle Stresses:

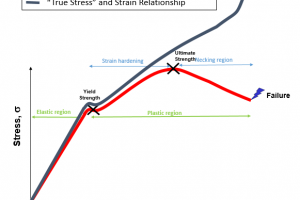
The principal stresses are the maximum and minimum normal stresses that act on a material at a particular point. These stresses are represented by σ_1 and σ_2, respectively. The principal stresses define the orientation of the principal planes, which are the planes where the shear stress is zero.

The principal stresses are significant because they provide essential information about the state of stress in a material or structure. They help determine whether a material is under tension or compression and also provide insights into its strength and failure behavior. By comparing the magnitudes and orientations of the principal stresses, engineers can evaluate the safety and stability of a structure.

Mohr's circle and principle stresses are particularly useful in analyzing complex stress states, such as those found in structures subjected to combined loading or in materials with anisotropic properties. They provide a graphical and intuitive approach to understanding stress distribution and allow engineers to make informed design decisions and assess the structural integrity of materials and components.